Hot Math Craft Posts


How To: Make a Sonobe Jasmine Dodecahedron
Math Craft admin Cory Poole posted instructions on How to Make a Cube, Octahedron & Icosahedron from Sonobe Units, plus some great complex models in his article, How to Make a Truncated Icosahedron, Pentakis Dodecahedron & More. These models use the standard sonobe unit and a coloured variant.

News: Fractal Pecan Pie
Following the pattern of fractal goodies, I found this great article on making a giant fractal pecan pie. Seems like you'll need some dedicated pecan pie enthusiasts (shouldn't be hard to find) to help you out!

MO MATH: Museum of Mathematics
The Museum of Mathematics, curated by George Hart, will be opening in 2012. Here are a few activities you can check out in the meantime.

News: 30 Square Sliding Modular Origami
Cory's post with instructions and templates Here's my first attempt at the 30 squares model. I needed to be a little bit more careful in the measuring and cutting as not everything matches up - but it is still a really pleasing shape.

How To: Make Fractal Cupcakes
Last post, we looked at fractal cookies based off of the recipe by Evil Mad Scientist Laboratories. In this post, we'll follow their recipe for fractal cupcakes based off of the Koch Snowflake, which we used previously to decorate pumpkins for Halloween.

News: An Octahedron Made with Sonobe Units
My first attempt with the sonobe unit! I'm now addicted. I'll be spending the holiday folding. Instructions here.

How To: Anybody Know How to Make This?
Via gurmeet.net

News: Origami Panda
So, this is a type of origami that is called golden venture origami. This type of origami is made of hundreds to thousands of little intersecting triangles. This took about 2-3 three hours, the picture is pretty bad because it was taken at school with a cell camera. But I hope this inspires you to make some of your own! This type of origami is in essence very easy to make but takes a lot of time and effort to make. However if you get really good at it, you can do it without even looking at ab...

News: A Compound of Two Cubes with a Minecraft theme
Compound of two cubes with a Minecraft theme.

News: DIY Crazy Paper Toy
This is just too cool. As soon as I saw this, I thought, "Math Craft!"

News: Orderly Tangle Earrings
I decided I would make those earrings I alluded to in Monday's Post on orderly tangles. I had to shrink the templates down so that the triangles are about 2 cm on a side. I used 110 lb cardstock and and painted them using metallic leafing paint in gold, silver, copper, and brass. I would put up a tutorial, except I think that this project would be too frustrating for most people. All I can suggest is that you make the orderly tangle of 4 triangles multiple times and just keep shrinking the si...

News: Tom Friedman's Twisted Math Art
Tom Friedman is one of my favorite artists. He's got a great sense of humor, and his work is meticulous and beautiful. He forays into Math Art, and from a partisan perspective, he seems to be inspired by mathematics, but the end results are more of a whimsical twist than a mathematically "correct" execution. But I could be totally wrong. Comment below and fill me in.

News: Math Craft Inspiration of the Week: Marble Binary Calculators & Other Mechanical Computers
Since today (11/11/11) is the last 6 digit binary date this century, I thought we should look at some kinetic binary calculators.

News: Modular Origami
Cory has posted some great picture of Father Magnus' intersecting cubes (the great man is holding one in his right hand) - well the above is what happens when five tetrahedra intersect. It is modular origami and made from just ten sheets of origami paper. technically in a folding sense it is easy - but putting it together is mind-warping



News: Curved Modular Octahedron
Each curved module replaces the equilateral triangle of a simple octahedron. Inspired and copied from Cory's post with original artwork by Richard Sweeney

News: Curves With Staight Lines
Much more complex than I had to make it- that's why I posted it. I think it looks cool...

News: The Unreasonable Beauty of Mathematics
Back in August, Scientific American posted a slideshow fitting for Math Craft. Click through to check out a slideshow depicting beauty found in mathematical structures—including a beautiful knot theory chart befitting of this week's project.

News: Beautiful Knot Sculptures
NYC based sculptor Meghan Forsyth created these beautiful knot sculptures in 2010. Can you identify which knots are depicted?

How To: Make Knot Sculptures from Soft Metals
In mathematics, a knot is a closed circle in a three-dimensional space that crosses itself multiple times. Since it is closed, it has no ends to tie, meaning you can't actually create such a knot. However, if you tie the ends together after you create a knot in the standard way, you will have something that is close to the mathematical description. In this post, we will explore the creation of mathematical knot sculputures using copper tubing and solid solder wire.

Math Craft Monday: Community Submissions (Plus Polyhedral Stellation)
It's another Monday, which means it's once again time to highlight some of the recent community submissions posted to the Math Craft corkboard. Additionally, I thought we'd take a look at the process of stellation and make some stellated polyhedra out of paper.Rachel Mansur of Giveaway Tuesdays posted a video from animator Cyriak Harris, which zooms into fractal hands, where each fingertip also has a hand and fingers. A few more details can be found here, as well as some other really cool pic...

News: Rotating Mirror Stellated Octahedron
The initial idea for this project was to use magnets in the tips of the stellated octahedron and the intersections of the metal rings for either suspension or even a sort of weightless rotation. This turned out to be a bit too ambitious considering I'm working with found mirror and hot glue. So instead, I scrapped the magnets and went with simply mounting it on a skateboard bearing so it can freely rotate and not be bound to the base.

How To: Carve Fractals and Stars on Pumpkins
Fractals and stars are two of the most beautiful and complicated-looking classes of geometric objects out there. We're going to explore these objects and how to carve them on a pumpkin. Unlike the last one on carving polyhedral pumpkins, where we used the entire pumpkin to carve a 3 dimensional shape, the pumkin carving in this post will involve two-dimensional images on a small part of the pumpkin's surface.
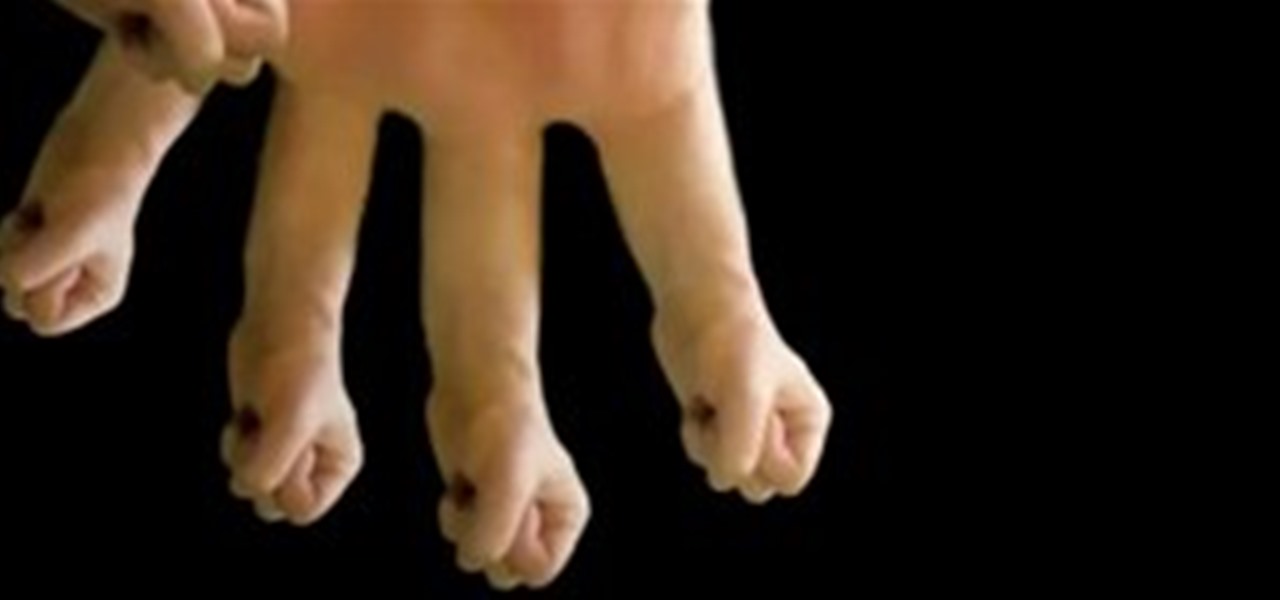
News: Cyriak Harris' Fractal Freakiness
I'm sure many of you have already seen this, but being Halloween and mathematically inspired, I thought I'd dig up an old favorite for those who may have missed it. Original post with quote from Cyriak here. More fractal hands: Tim Hawkinson's "Fruit" Series

How To: Carve Polyhedral Pumpkins
Halloween is coming up, so many of you may have a need or desire to carve a pumpkin and turn it into a Jack O' Lantern. This week we are going to explore carving our pumpkins into interesting geometric shapes. In this post, we will carve the pumpkins into spherical versions of polyhedra, and in Thursday's post we will carve 2 dimensional stars and some simple fractal designs into the pumpkins.

Math Craft Monday: Community Submissions (Plus Tiling with Coins)
It's Monday, and once again it's time to highlight some of the community submissions posted to the Math Craft corkboard. In addition, I thought we'd take a look at having fun with the geometrical properties of polygons and circles by using one of the best circles I know, the penny.

News: Beyond Infinity Immersive Installation
Combine a bunch of fractal objects with mirrors and and lights and the ability to walk around inside and you get something like the image below.

News: The Venn Piagram
Someone made this awesome pie, or is it two, in the shape of the standard two circle Venn Diagram.

Penny Polyhedra: Cube
Not the best picture, but will do. More will be coming!

News: Torolf Sauermann
A beautiful object by artist Torolf Sauermann; see more of his math art here.
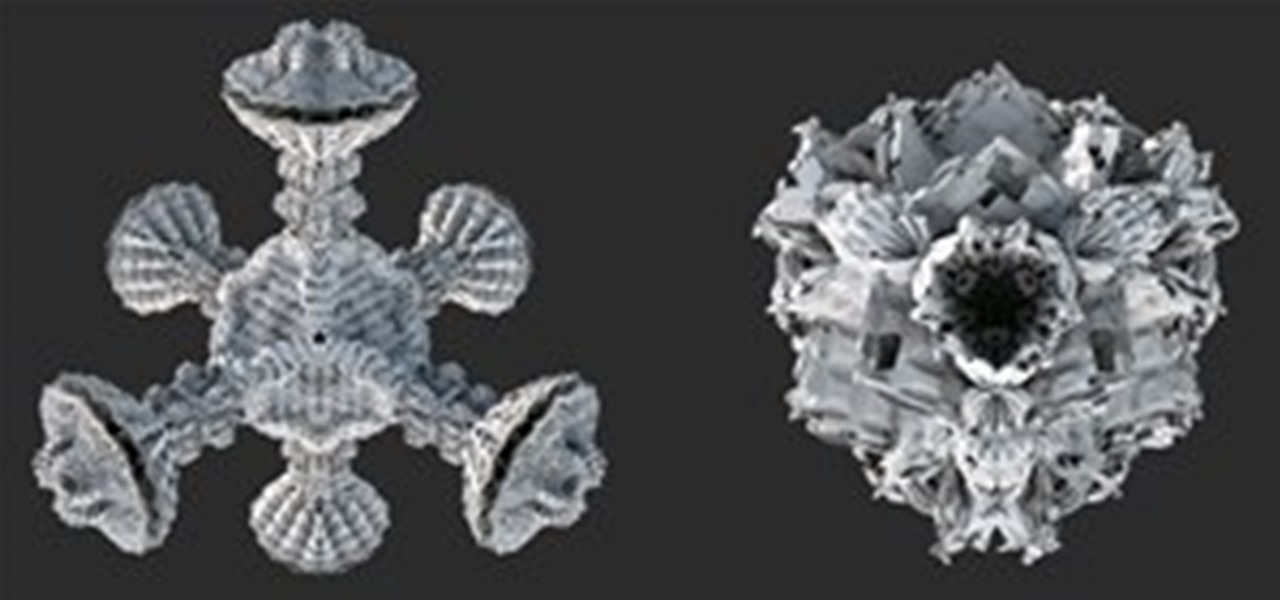
News: The Platonic Solids Get Trippy
If you haven't participated in this week's Math Craft project on the platonic solids, maybe this will inspire you to do so.

A Kit-of-No-Parts: "Crafting" Electronics at MIT
I recently came across this amazing MIT media lab site, Kit-of-No-Parts. Though not directly related to the content Cory has been posting, it is an interesting "craft" approach to technology/science. The site was created as documentation of a student's thesis work in the High-Low Tech research group at the MIT Media Lab:

News: Math Craft Inspiration of the Week: The Curved Geometric Paper Sculptures of Richard Sweeney
Richard Sweeney is an incredible artist whose body of work consists mainly of sculptures made from paper. His art is often related to origami, and much of his work is related to geometrical forms. I personally really love his modular forms in paper. Many of them are based off of the platonic solids, which have been discussed in previous posts this week. Below are a small number of his sculptures, which are very geometric in nature.

News: Icosahedron + Cube!
It makes a nice little snow man hehe

News: Albrecht Dürer, the Father of Polyhedral Nets
Did you know that you can "write" in polyhedra? I just stumbled across a $24.99 font called Divina Proportione. Created by Brazilian graphic designer Paulo W, the typeface is constructed with beautiful geometric renderings by the famous Renaissance printmaker Albrecht Dürer.

News: Watch Out Nemo! Fish-Shaped Gears
I built this the other day from those weird gear plans from Clayton Boyer.







